Cookie Policy

Welcome to SR-NIEL. This site uses cookies. Read our policy.

Learn more
Logo HelMod small

SR-NIEL – 7

Screened Relativistic (SR) Treatment for NIEL Dose

Nuclear and Electronic Stopping Power Calculator

(version 10.20)

 Proton High AMS02 small

SR-NIEL Website

ASIF Related Websites

Statistics

  • Articles View Hits 1122104

  • Requests to
    sr-niel calculators
    76082
    daily updated

Who's Online

We have 90 guests and no members online

Related Links

Website Search

Probability in cm^2/g of energetic recoil nuclei caused by electrons

Form Factor Model:
Target Materials
Target Selection:
N° Elements:
Target Material Z Stoichiometric Index or Element Fraction
Energy ranges
Minimum Energy: [MeV]
Maximum Energy: [MeV]
Recoil Energy Eener: [MeV]
Additional Energies (optional): [MeV]


EXAMPLE:
Energetic nuclear recoil probability for Electrons in Silicon with Exponential form factor and Eener=0.1 MeV:

NOTE:
  • The Probability for an incoming electron to generate a recoil nuclei above the requested Eener value is derived by

    (total probability in cm2g-1) x (absorber density in g cm-3) x (traversed thickness in cm),

    where the "total probability in cm2g-1" is obtained by the web calculator, absorber density and traversed thickness are defined by the user.
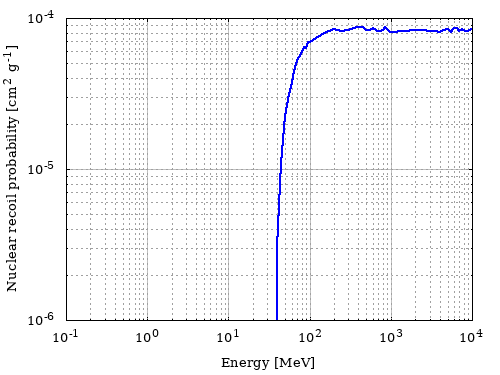
    Eener is the the lower limit of recoil energy (for explanation see Energetic Nuclear Recoil Page)
  • The Probability depends on the energy of the incoming particle.
  • In the present treatment, screening effects are factorized in the expression for the differantial cross section (Boschini et al. (2011)). However, it has to be remarked - as derived by Zeitler and Olsen (1956) - that for electron energies above 200 keV the overlap of spin and screening effects is small for all elements and for all energies; for lower energies the overlapping of the spin and screening effects may be appreciable for heavy elements and large angles.
  • User can suggest an "ad hoc" form factor by contacting us using This email address is being protected from spambots. You need JavaScript enabled to view it. . In fact (see discussion in Boschini et al. (2011)), a further work is likely to be needed for the most suited parametrization of the nuclear form factor, particularly for high-Z naterial.
  • Energetic nuclear recoil probability for compounds can be determined by means of Bragg's additivity rule, i.e., the overall Probability in units of cm2/g is obtained as a weighted sum in which each material contributes proportionally to the fraction of its atomic weight. An example for selection, with water (H2O) as target, follows:

    or equivalently

  • The Total Cross Section is not affected by the Form Factor term. This because the Differential Cross Section is larger by several orders of magnitude with respect to the corresponding values at the very low angles, where the form factor is relevant. The effect of different form factors on differential cross section for 184 MeV electrons in In (Z=49) is in the following images, with the same experimental data set from B.Hahn et al. (1956). Our calculation is on the left and the graph from Fernandez-Varea et al. (1993) is on the right.:

    However the transferred energy is several order of magnitude larger at high scattering angles.

    The overall results is that the NIEL and the Nuclear Stopping power (thus, the Recoil Probability) are affected by the form factor. Numerical results for 183 MeV electrons in In are presented in the following table:

    • Details
    Hits: 3764