Coulomb Interactions of Electrons on Screened Nuclei

 

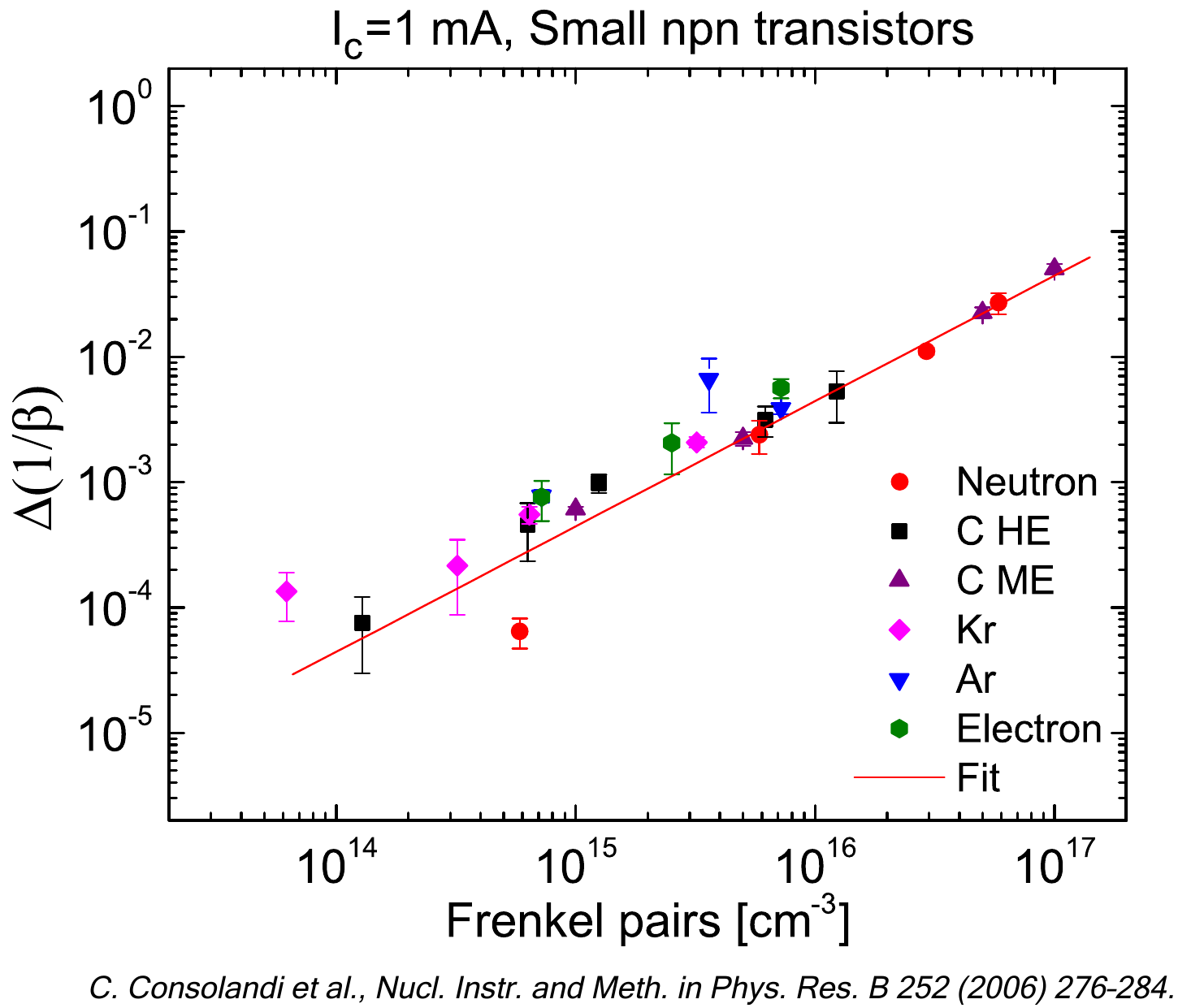
The electron–nucleus scattering used in the sr-niel approach is treated, for instance, in Chapter 2 and of [Leroy and Rancoita (2016)] (see also [Boschini et al. (2012), Boschini et al. (2013)] and references therein). In this framework, the Mott differential cross section (MDCS), dσ_sc,F,CoM^Mott(T)∕dT dT, is derived and employed accounting for effects due to the screened Coulomb fields, finite sizes and rest masses of nuclei. 

The scattering of electrons by unscreened atomic nuclei was treated by Mott (1929) (see also Sections 4–4.5 in Chapter IX of [Mott and Massey (1965)]) extending a method of Wentzel (1927) (see also [Born (1926)]) and including effects related to the spin of electrons. Wentzel’s method was dealing with incident and scattered waves on point-like nuclei. The differential cross section (DCS) - the so-called Mott (unscreened) differential cross section (MDCS) - was expressed by Mott (1929) as two conditionally convergent infinite series in terms of Legendre expansions (see also [Bartlett and Watson (1940)] and Equation 46 in Chapter IX of [Mott and Massey (1965)]). In Mott–Wentzel treatment, the scattering occurs on a field of force generating a radially dependent Coulomb - unscreened [screened] in Mott (1929) [Wentzel (1927)] - potential. Mott equation - computed using Darwin’s solution to the Dirac equation - is also referred to as an exact formula for the differential cross section, because no Born approximation of any order is used in its determination. 

Furthermore, the MDCS was derived in the laboratory reference system for infinitely heavy nuclei initially at rest with negligible spin effects and must be numerically evaluated for any specific nuclear target. Effects related to the recoil and finite rest mass of the target nucleus (M) were neglected. Thus, in this framework the total energy of electrons has to be smaller or much smaller than Mc².

It has to be remarked that Mott’s treatment of collisions of fast electrons with atoms - accounting for screening effects - involves the knowledge of the wave function of the atom and uses the first Born approximation (e.g., see Sections 2–5 of Chapter XVI of [Mott and Massey (1965)]); thus, as discussed by many authors (for instance, see [Idoeta and Legarda (1992), Lijian, Quing and Zhengming (1995), Boschini et al. (2012), Boschini et al. (2013)] and also references therein), in most cases the computation of the cross section depends on the application of numerical methods (see a further discussion in Sect. 2.4.2 in [Leroy and Rancoita (2016)]). Particularly, in calculations for electron transport in materials or in the determination of induced radiation damage due to atomic displacements resulting from Coulomb interaction on nuclei, this treatment may require an excessive time-consuming procedure for accounting the effect of nuclear screening by atomic electrons. 

In practice, for the above mentioned calculations a factorization of the elastic screened cross section is often employed. It involves the unscreened differential cross section on point-like nuclei and a factor which takes into account the screening of the nuclear charge by the atomic electrons. Expressions for this term - which is also employed in the treatment of nucleus–nucleus interactions - were derived and discussed by many authors. Furthermore, in electron scattering on nuclei above 10 MeV, as discussed by Fernandez-Varea, Mayol and Salvat (1993a) (see also [Boschini et al. (2012)] and references therein), the effects due to the finite nuclear size have to be taken into account and are usually expressed by a multiplicative term, the so-called nuclear form factor.

Among the spherically symmetric form factors treated in literature, one finds those for i) the exponential charge d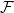istribution (_exp) (e.g., see Equation (6) of [Butkevick (2002)], Equation (93) at page 252 of [Hofstadter (1957)] and references therein), ii) the Gaussian charge distribution (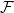_gau) (e.g., see Equation (6) of [Butkevick (2002)] and references therein) and, finally, iii) the uniform–uniform folded charge distribution over spheres with different radii (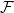_u) (e.g., see Equation (22) of [Fernandez-Varea et al. (1993a)], [Helm (1956)] and references therein). 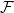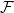The above mentioned form factors _exp, _gau and 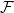_u are expressed as:

(1)

(e.g., see Equation (6) of [Butkevick (2002)]), where r_n is the nuclear radius, approximated by

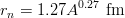(2)

with A the atomic weight (e.g., see Equation (7) of [Butkevick (2002)]),

(3)

(e.g., see Equation (6) of [Butkevick (2002)]) and, finally,

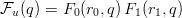(4)

with q the value of the momentum transfer and

(e.g., see Equations (19–22) in [Fernandez-Varea et al. (1993a)]). One has to note that, from deuterium up to heavy nuclei (like Pb and U), Eq. (2) provides a values of r_n usually in agreement within 5% with the root mean square radius of the nuclear charge distribution, (see, for instance, in Table 1 of [De Vries, De Jager and De Vries (1987)]). 

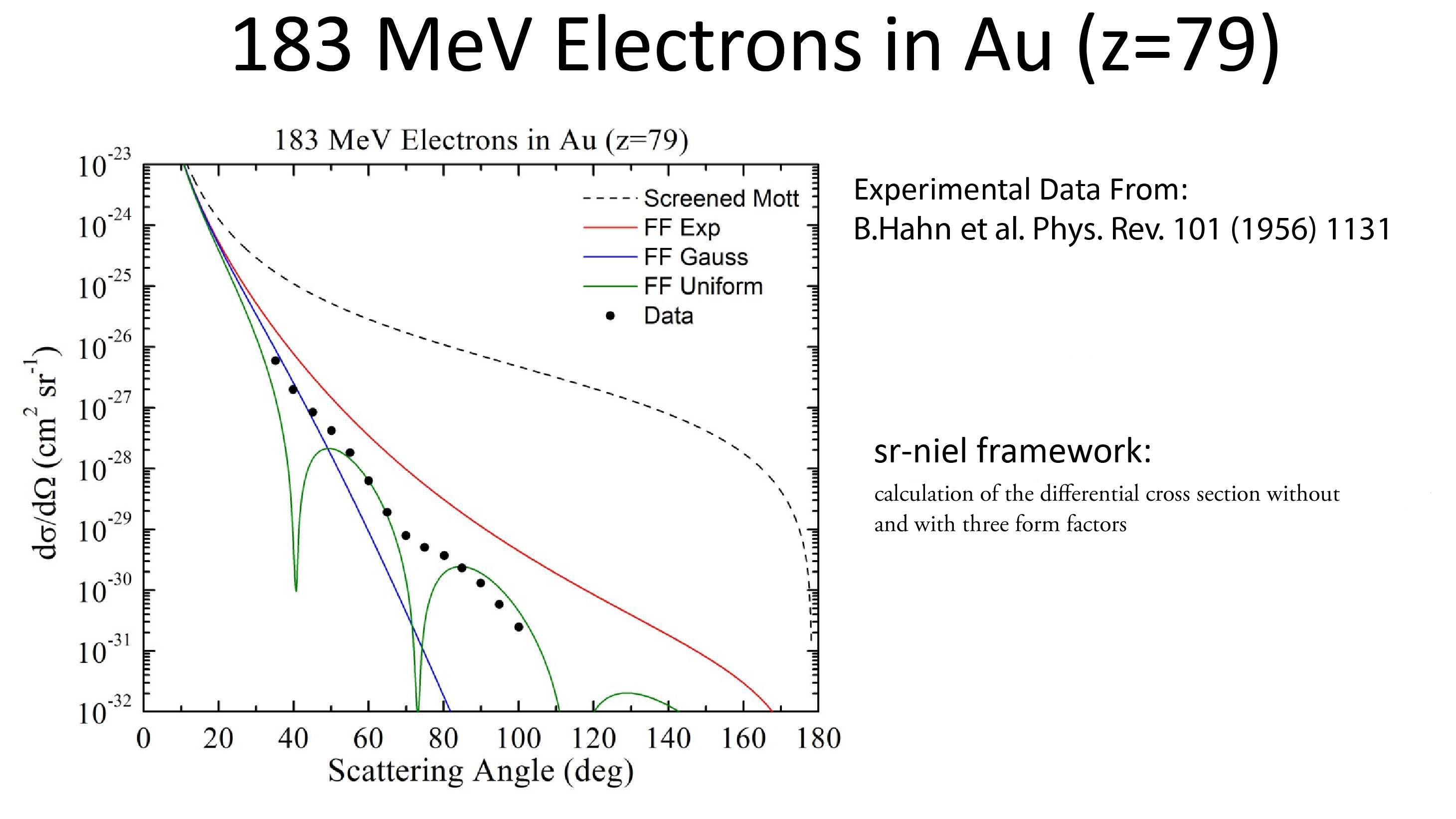
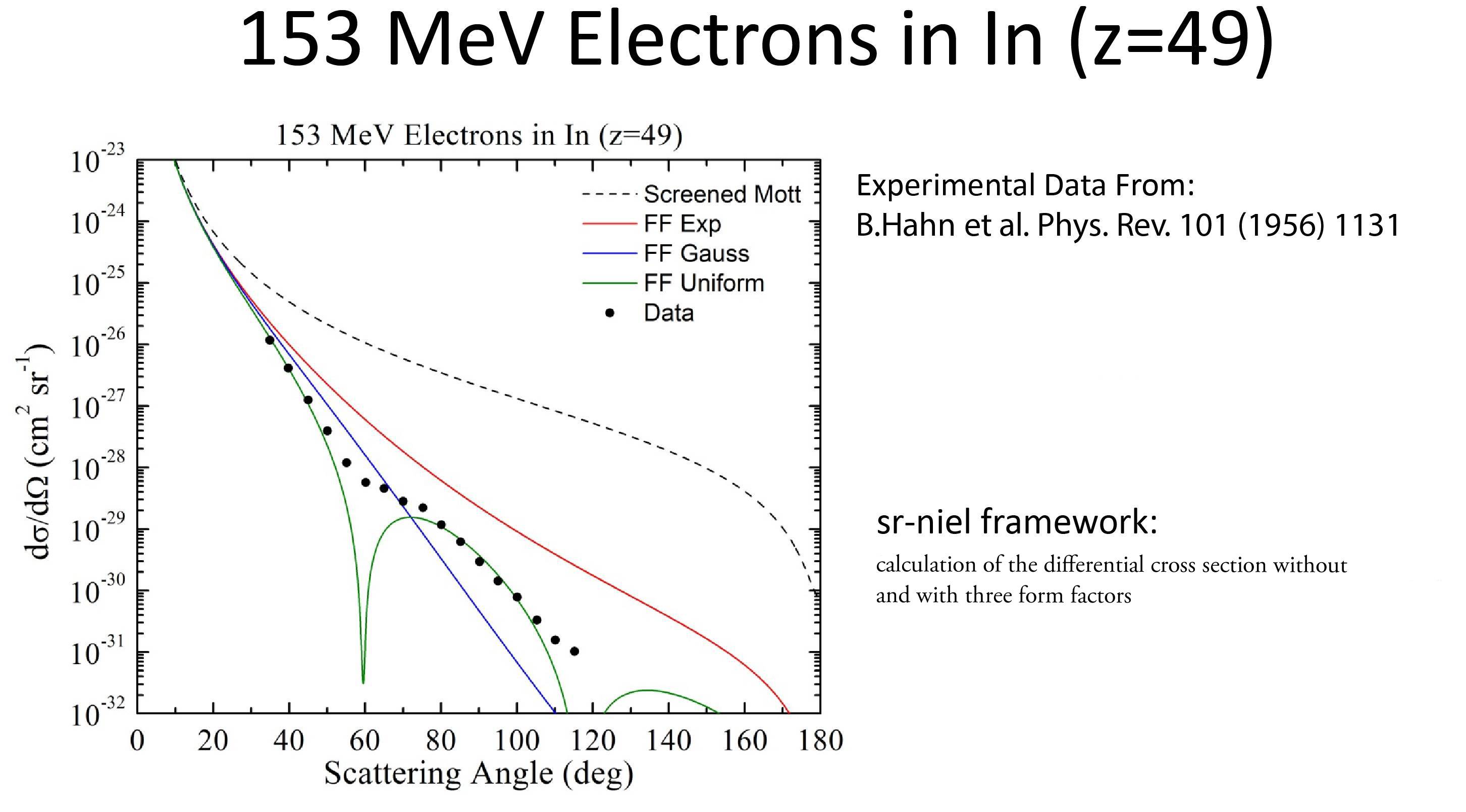
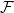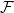Furthermore, one can remark (e.g., see footnote at page 186 of [Leroy and Rancoita (2016)]) that the Mott differential cross sections - calculated using sr-treatment with _exp, _gau and 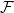_u form factors - were capable to describe the large angle decreasing found in experimental data of electron scattering on He (at 2.091 and 4.048 GeV from [Camsonne et al. (2014)]), C (at 375 and 750 MeV from [Sick and McCarthy (1970)]), O (at 375 and 750 MeV from [Sick and McCarthy (1970)]), Al (at 250 and 500 MeV from [Li and Yearian (1974)]), Si (at 250 and 500 MeV from [Li and Yearian (1974)]), In (at 153 and183 MeV from [Hahn et al. (1956)]), Au (at 153 and183 MeV from [Hahn et al. (1956)]) - slightly favoured were 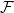_gau or 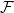_u form factors -; as expected, the data largely disagreed with the calculated values using |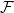|² = 1. Furthermore, using |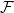|² = 1 the stopping powers resulted larger from about 13.8% for Al at 250 MeV up to about 28.3% for Au at 183 MeV with respect to those derived with 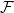_exp. Finally, it is worth to note that the Mott cross section resulted marginally affected by the form factor, because electron scatterings at large angles are extremely improbable. at large energies replacing 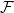_exp with 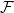_gau or 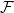_u. However, a further study is needed to determine a most suited parametrization of the nuclear form factor particularly for high-Z materials (e.g., see [Nagarajan and Wang (1974), Duda, Kemper and Gondolo (2007), Jentschura and Serbo (2009)]).

Approximate expressions for the Mott (unscreened) differential cross section were derived as early as in the 1940s and 1950s (e.g., see [Bartlett and Watson (1940), McKinley and Feshbach (1948), Feshbach (1952), Curr (1955), Doggett and Spencer (1956)), Sherman (1956)]).

Idoeta and Legarda (1992) (as suggested, for instance, in [Sherman (1956)]) evaluated the MDCS exploiting recursion relationships of the gamma functions showing that the ratio - appearing in the MDCS - fulfills the condition for the application of the Stirling’s formula. In addition, they applied the trasformation of Yennie, Ravenhall and Wilson (1954) to the infinite series of Legendre polynomials. Finally, they obtained tabulated values for electrons and positrons scattering on a few nuclei with kinetic energies from 5 keV and 10 MeV and a maximum error of less than 10^-3 %. Moreover, they discussed the good agreement of their results with those previously found - within the sensitivity of the used approximations - in [McKinley and Feshbach (1948), Curr (1955), Yadav (1955), Motz, Olsen and Koch (1964), Doggett and Spencer (1956)), Sherman (1956)].

Subsequently, Lijian, Quing and Zhengming (1995) developed a fitting procedure for the numerical values determined following the approach of Idoeta and Legarda (1992), then expressing the ratio (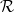^Mott) of the MDCS to Rutherford differential cross section (RDCS) as an analytical formula depending on 30 parameters with a maximum error of less than 1 % only for electrons with kinetic energies from 1 keV up to 900 MeV. Above 900 MeV, no further energy dependence was exhibited by the parameters. These parameters depend on the nuclear target and were tabulated for nuclei with Z up to 90. More recently, Boschini and collaborators (2013) also discussed an improved numerical approach - employed in the current sr-niel framework and web-calculators - for determining the ratio, ^Mott, of the unscreened MDCS with respect to Rutherford’s formula. These results were compared and found in excellent agreement with those from Idoeta and Legarda (1992). Moreover, the calculated numerical values of ^Mott - obtained from that improved numerical solution - were used to provide an interpolated practical expression for both electrons and positrons scattering on nuclei with 1 ≤ Z ≤ 118 in the kinetic-energy range from 1 keV up to 900 MeV. The latter expression was also compared with that found previously - when available and usable - for electrons (on nuclei with 1 ≤ Z ≤ 90) by Lijian, Quing and Zhengming (1995). The two expressions exhibited a very good agreement for low and high-Z nuclei.

Furthermore, Boschini and collaborators (2012) extended the treatment of the electron–nucleus interaction based on the Mott differential cross section to account for effects due to screened Coulomb potentials, finite sizes and finite rest masses of nuclei for electrons above 200 keV and up to ultra high energies. This extended treatment allows one to determine both the total and differential cross sections, thus, subsequently to calculate the resulting nuclear and non-ionizing stopping powers (or non-ionizing energy-loss, NIEL, e.g., see Chapter 7 of [Leroy and Rancoita (2016)]). 

In the sr-niel framework, the unscreened and screened Coulomb electron–nucleus scattering are dealt following the approach of Boschini and collaborators (2012, 2013). The unscreened MDCS is discussed in Sects. 2.4.1–2.4.1.2 of [Leroy and Rancoita (2016)] in which the analytical approximate equation from [McKinley and Feshbach (1948)] and the interpolated expressions from [Lijian, Quing and Zhengming (1995), Boschini et al. (2013)] are discussed for the scattering on a target nucleus with a rest mass much larger than the mass corresponding to the (total) energy (E) of the incoming particle. In addition, the Coulomb elastic scattering with screened nuclear potentials and effects due to both the finite nuclear size and rest mass are discussed in Sects. 2.4.2-2.4.2.2 of [Leroy and Rancoita (2016)]. While, the calculation of the Coulomb nuclear stopping power of electrons in materials is treated in Sect. 2.4.3 of [Leroy and Rancoita (2016)].

Finally, it has to be remarked that the currently described treatment of the MDCS for elastic scattering on nuclei was implemented into the current Geant4 distribution (e.g., see [Agostinelli et al. (2003), Geant4: Class G4eSingleScatteringModel (2015)]) (see, also, the discussion in [Boschini et al. (2012)] and references therein).

References