SR-Treatment and Calculation of Nuclear (and Mass) Stopping Power for Elements and Compounds

Screened Relativistic Nuclear Stopping Power for Electrons, Protons, Ligh- and Heavy-Ions

For a charged particle passing through a medium, the stopping power (S) of the material is numerically equal to the loss of energy per unit path length experienced by the particle in the absorber (i.e., the energy loss ). In case the scattering occurs on nuclei, the stopping power is termed nuclear stopping power ( ) and, thus, it is the energy lost [] by the incident particle, caused by such interactions, i.e.,

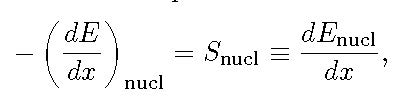
where the minus sign indicates that the incoming particle loses part of its energy (e.g., see discussions in Sects. 2.1.4, 2.1.4.2 of [Leroy and Rancoita (2011)], Sect. 1.3.2 of [Leroy and Rancoita (2012)] and Chapter 2  [Leroy and Rancoita (2016)]).

For instance, in case of (elastic) Coulomb scattering of electrons or protons or ions on nuclei, the nuclear stopping power can be calculated as

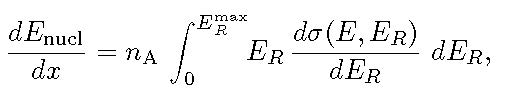
where

is the number of atoms per cm³ in the absorber,  and A are the density and atomic weight of the medium, respectively; N is the Avogadro constant, E is the kinetic energy of the incoming particle; E_R and E_R ^max are the recoil kinetic energy and the maximum energy transferred to the recoil nucleus, respectively; finally,  is the differential cross section for elastic Coulomb scattering for electrons or protons or ions on nuclei. Furthermore, the energy lost by the incoming particle can be expressed in units of MeV cm²/g as

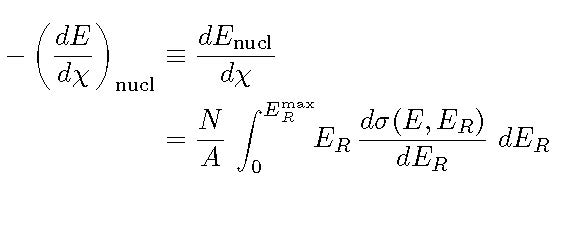
with , ρ_Athe absorber density in g/cm³ and  the nuclear mass-stopping power.

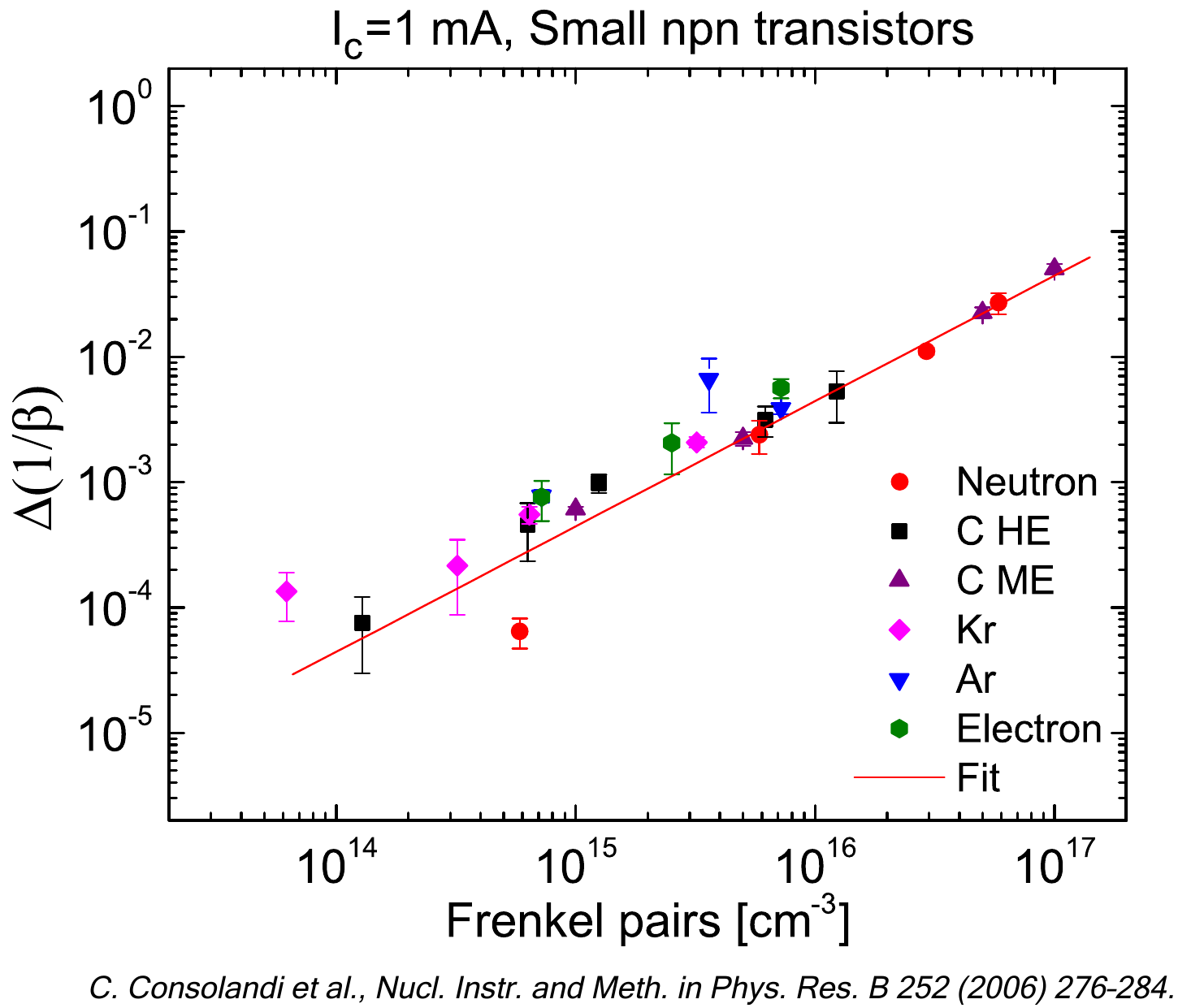
In the framework of the screened relativistic treatment for nuclear elastic scattering, the values of the nuclear mass stopping power in units of MeV cm²/g for electrons are obtained using the Mott differential cross section [Mott (1929)] of electrons on nuclei calculated from the practical expression discussed in [Boschini et al. (2013)] (see also [Linjian et al. (1995)]) and accounting for the effects due to both screened nuclear potentials and form factors discussed in [Boschini et al. (2012)] and Sects. 1.3.1-1.3.3 of [Leroy and Rancoita (2012)]  (see also references therein). Hereafter, (i.e., see Chapter 2 in [Leroy and Rancoita (2016)]) is reported the discussion about the currently implemented form factors in this calculation of the nuclear stopping power and screened relativistic NIEL.

We can remark (see footnote at page 186 of [Leroy and Rancoita (2016)]) that:
"The Mott differential cross sections - calculated using Eqs.(1.97, 2.273) and F_exp, F_gau and F_u form factors - were capable to describe the large angle decreasing found in experimental data of electron scattering on He (at 2.091 and 4.048GeV from [Camsonne et al. (2014)]), C (at 375 and 750MeV from [Sick and McCarthy (1970)]), O (at 375 and 750MeV from [Sick and McCarthy (1970)]), Al (at 250 and 500MeV from [Li and Yearian (1974)]), Si (at 250 and 500MeV from [Li and Yearian (1974)]), In (at 153 and183MeV from [Hahn et al. (1956)]), Au (at 153 and 183MeV from [Hahn et al. (1956)]) - slightly favoured were F_gau or F_u form factors -; as expected, the data largely disagreed with the calculated values using |F|² = 1. Furthermore, using |F|² = 1 the stopping powers resulted larger from about 13.8% for Al at 250MeV up to about 28.3% for Au at 183MeV with respect to those derived with F_exp. Finally, it is worth to note that the Mott cross section resulted marginally affected by the form factor, because electron scatterings at large angles are extremely improbable."

The nuclear stopping power for protons and nuclei above 50 keV/nucleon is obtained using the elastic cross section derived from treatment of the nucleus-nucleus screened Coulomb scattering discussed in [Boschini et al. (2011)] and Sect. 2.1.4.2 in [Leroy and Rancoita (2011)] (see also [Fernandez-Varea et al. (1993),Butkevick (2002), Boschini et al. (2010)]). That treatment allows one to deal with a scattering occurring up to relativistic energies.

For energies lower than 50-200 keV/nucleon, the scattering of protons and screened nuclei can be treated using the 4-terms analytical approximation of the ZBL cross section derived by Messenger et al. (2004) [see Eqs. (1--3, 15) and also references therein].

In literature, nuclear mass stopping powers for compounds were usually determined by means of Bragg's additivity rule as, for instance, those ones availabe from and tabulated for the commonly employed non-relativistic treatments of [ICRUM (1993)] and SRIM  (e.g., see this webpage and additionally here). In this way, for a compund the overall nuclear mass stopping power in units of MeV cm²/g is obtained as a weighted sum in which each material contributes proportionally to the fraction of its atomic weight. For instance, in case of a GaAs medium ones obtains (e.g.,, see [Jun et al. (2009)] and Eq. (2.20) at page 15 in [ICRUM (1993)]):

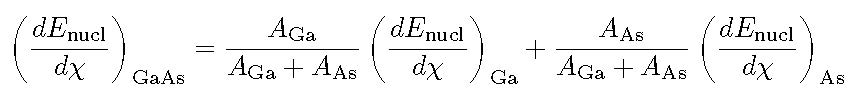
where  are the nuclear mass stopping power (in units of MeV cm²/g) and the atomic weight of Gallium [Arsenic], respectively. It has to be remarked that Bragg's additivity rule was established for the energy loss mechanism dominated (by orders of magnitude) by that resulting in ionization energy deposition (e.g., Sect. 2.1.2 of [Leroy and Rancoita (2016)],[ICRUM (1993)] and further discussions about its acuracy at this SRIM webpage). Detailed experimental studies of Bragg's additivity rule started in 1960s. 
Finally, the screened relativistic treatments for electrons [Geant4: Class G4eSingleScatteringModel (2014)], protons and ions [Geant4: Class G4IonCoulombScatteringModel (2014)] are inluded in Geant4 simulation code. The complete treatment can be found in Chapers 2, 7 and 8 of [Leroy and Rancoita (2016)].

References

M.J. Boschini, C. Consolandi, M. Gervasi, S.Giani, D.Grandi, V. Ivanchenko and P.G. Rancoita, (2010), Geant4-based application development for NIEL calculation in the Space Radiation Environment, Proc. of the 11th ICATPP Conference, October 5-9 2009, Villa Olmo, Como, Italy, World Scientific, Singapore, 698-708, IBSN: 10-981-4307-51-3;http://www.worldscientific.com/doi/pdf/10.1142/9789814307529_0113 .

M.J. Boschini, C. Consolandi, M. Gervasi, S. Giani, D. Grandi, V. Ivantchenko, S. Pensotti, P.G. Rancoita, M. Tacconi, (2011), Nuclear and Non-Ionizing Energy-Loss for Coulomb Scattered Particle from Low Energy up to relativistic regime in Space Radiation Environment, Proc. of the 12th ICATPP Conference, October 7-8 2010, Villa Olmo, Como, Italy, World Scientific, Singapore, 9-23, IBSN: 978-981-4329-02-6; http://www.worldscientific.com/doi/pdf/10.1142/9789814329033_0002 .

M.J. Boschini, C. Consolandi, M. Gervasi, S. Giani, D. Grandi, V. Ivanchenko, P. Nieminem, S. Pensotti, P.G. Rancoita and M. Tacconi, (2012), Nuclear and Non-Ionizing Energy-Loss of electrons with low and relativistic energies in materials and space environment, Proc. of the 13th ICATPP Conference, October 3-7 2011, Villa Olmo, Como, Italy, World Scientific, Singapore, 961-982, IBSN: 978-981-4405-06-5;http://www.worldscientific.com/doi/pdf/10.1142/9789814405072_0147 .

M.J. Boschini, C. Consolandi, M. Gervasi, S. Giani, D. Grandi, V. Ivanchenko, P. Nieminem, S. Pensotti, P.G. Rancoita, M. Tacconi, (2013), An expression for the Mott cross section of electrons and positrons on nuclei with Z up to 118, Rad. Phys. Chem. 90, 39-66; doi: 10.1016/j.radphyschem.2013.04.020.

A.V. Butkevick (2002), Nucl. Instr. and Meth. in Phys. Res. A 488, 282-294.

H. De Vries,C.W. De Jager and C. De Vries (1987), Atomic Data and Nuclear Data Tables 36, 495.

Geant4: Class G4IonCoulombScatteringModel~(2014). Class Description: Single Scattering Model for protons, alpha and heavy Ions (Ref.~Boschini et al. 2011), Software Reference Manual. Source Code Version: Geant4 10.0 (December 2013) avaliable at the website
http://www-geant4.kek.jp/LXR
See also Sections~6.5--6.5.7 of Physics Reference Manual for version 10.0 available at the website:
http://geant4.cern.ch/support/userdocuments.shtml

Geant4: Class G4eSingleScatteringModel (2014) . Class Description: single scattering interaction of  electrons on nuclei (Ref.~Boschini et al. 2012), Software Reference Manual . Source Code Version: Geant4 10.0 (December 2013) avaliable at the website 
http://www-geant4.kek.jp/LXR 
See also Sections~6.7--6.8 of Physics Reference Manual for version 10.0 available at the website: 
http://geant4.cern.ch/support/userdocuments.shtml

ICRUM - International Commission on Radiation Units and Measurements - (1993), Stopping Powers and Ranges for Protons and Alpha Particles, ICRU Report 49, Bethesda, MD.

I. Jun et al. (2003), IEEE Trans. on Nucl. Sci 50, 1924-1928.

I. Jun et al. (2009), IEEE Trans. on Nucl. Sci. 56, 3229--3235

C. Leroy and P.G. Rancoita (2011),  Principles of Radiation Interaction in Matter and Detection - 3rd Edition -,World Scientific, Singapore, ISBN-978-981-4360-51-7;http://www.worldscientific.com/worldscibooks/10.1142/8200 .

C. Leroy and P.G. Rancoita (2012), Silicon Solid State Devices and Radiation Detection, World Scientific, Singapore, ISBN-978-981-4390-0-0;http://www.worldscientific.com/worldscibooks/10.1142/8383 

.

C. Leroy and P.G. Rancoita (2016), Principles of Radiation Interaction in Matter and Detection - 4th Edition -, World Scientific. Singapore, ISBN-978-981-4603-18-8 (printed); ISBN.978-981-4603-19-5 (ebook). https://www.worldscientific.com/worldscibooks/10.1142/9167#t=aboutBook; it is also partially accessible via google books.

T. Linjian, H. Quing and L. Zhengming (1995), Radiat. Phys. Chem. 45, 235-245.

S.R. Messenger et al. (2004), IEEE Trans. on Nucl. Sci. 51, 2846-2850.

N.F. Mott (1929), Proc. Roy. Soc. A 124, 425-442; A 135 (1932), 429-458.

J.M. Fernandez-Varea et al. (1993), Nucl. Instr. and Meth. in Phys. Res. B 73, 447–473. 

Helm, R.H. (1956). Phys. Rev. 104, 1466-1475.

Hofstadter, R. (1957). Ann. Rev. Nucl. Sci. 7, 231.

SRIM - The Stopping and Range of Ions in Matter - SRIM-2013

E. Zeitler and A. Olsen, Phys. Rev. 136 (1956), A1546-A1552. 

J.M. Fernandez-Varea, R. Mayol and F. Salvat, Nucl. Instr. and Meth. in Phys. Res. B82, (1993) 39-45

B.Hahn et al. Phys. Rev. 101 (1956) 1131